Derivative of Tanx is Sec2x
Are you curious about the Sec2x, a derivative of tanx? If you are, read this article. The information will help you understand how to calculate tanx. Here’s an example. In a question like “what is the derivative of tanx?”, the answer is the product formula f(x). Then, you can use the chain rule to get the derivative of tanx.
Sec2x
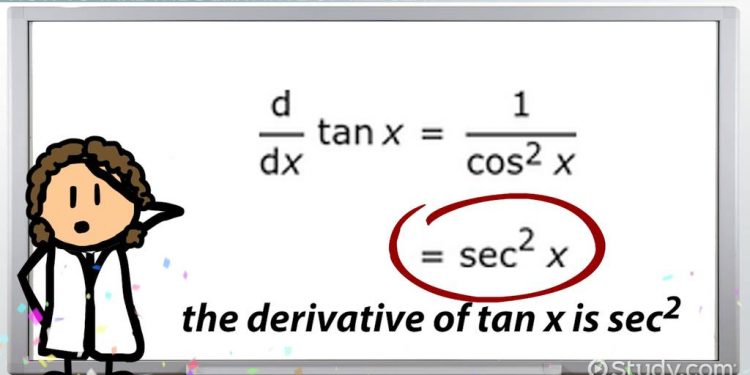
If you have ever written the formula dx = tanx, then you’ve likely heard that the derivative of Tanx is Sec2x. In fact, the trig identity isn’t exactly the same as the formula, as tanx has two meanings. The former is an expression that measures the inverse of the other variable, while the latter is an equation that measures the ratio of two variables. The trig identity of Tanx is Tx.
Essentially, Sec2x is the difference between the tan X function and the cosecant function. The cosecant function is the reciprocal of Tanx. The tan x function is continuous on the interval -p/2, but it has infinite points of discontinuity. The derivative of Sec2x, therefore, is f(x).
The second step in calculating the derivative of Sec2x is to find the product rule. This rule is used to determine the difference between the two derivatives of Sec2x. The product rule can be applied to the derivative of Sec2x, and it can be used to differentiate expressions in x. It is also useful in finding the derivative of a function in x. The product rule is often used when determining the derivative of Sec2x.
Sec2x = tanx
We all know that Sec2x=tanx is one of the most useful security programs on the market. However, many people are still confused about this formula. To help you out, here are some explanations. First, let’s define what Sec2x is. This is a mathematical equation whose solution depends on a specific value of dx. Besides determining the value of dx, Sec2x can also be used as a determinant in the tan system.
Sec2x = sec2x
You’ve probably seen the formula for the derivative of tanx or sec2x, but what does this mean? Basically, it means that the value of x is the derivative of tanx. It’s easy to prove this statement – just draw the function on a piece of graph paper and read it. If you don’t understand the equation, you can look up some other methods to differentiate expressions in x.
The derivative of a single-variable function is the slope of its tangent line, the closest linear approximation of the function near the input value. In mathematics, the derivative is also known as instantaneous rate of change. Using this equation, we can deduce that sec2x = derivative of tanx. The first term of the equation is kept as is and the second is the derivative of sec2x.
Similarly, the sec2x derivative of tanx is the cosine of x. This derivative has a vertical asymptote at -p/2 and is undefined at -dfracpi2. This is because it has infinite points of discontinuity. However, it has a limit, which is the simplified difference quotient. We can use the simplified difference quotient to determine how tanx is related to h.
Then, to differentiate tanx, we need to use the formula for first-principles differentiation. This step involves trig identities, simplifying, substituting, and combing terms. We can also use log laws, which are essentially a derivative of tanx. Finally, we can find the derivative of tanx in algebra. This process requires a basic understanding of algebra and trigonometric identities.